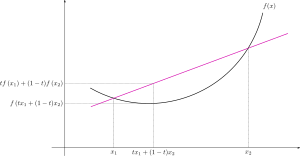
Convex function

Convex function on an interval.

A function (in black) is convex if and only if the region above its graph (in green) is a convex set.

In mathematics, a convex function is any function with value of the weighted average of 2 points is less than or equal to the weighted average of the function at those points. Also, a function is convex if and only if its epigraph is a convex set.

Examples

Three examples are:

• A line: [math]\displaystyle{ f(x) = x }[/math]
• A parabola: [math]\displaystyle{ f(x) = x^2 }[/math]
• Absolute value: [math]\displaystyle{ f(x) = |x| }[/math]

Convex Function Media

• 

  A graph of the bivariate convex function x2 + xy + y2.

• 

  In a convex function, it is impossible to connect two different points on the curve and cross the curve with the line.

• Visualizing a convex function and Jensen's Inequality