Orbital hybridization
In chemistry, hybridization (or hybridisation) is the concept of mixing atomic orbitals into new hybrid orbitals suitable for the pairing of electrons to form chemical bonds in valence bond theory. Hybrid orbitals are very useful in the explanation of molecular geometry and atomic bonding properties. [note 1]
Historical development
Chemist Linus Pauling first used hybridization theory to explain the structure of molecules such as methane (CH4).[1] This concept was developed for such simple chemical systems. But the approach was later applied more widely. Today, chemists use it to explain the structures of organic compounds.
Orbitals represent how electrons behave within molecules. In the case of simple hybridization, this approximation is based on atomic orbitals. Chemists use the atomic orbitals of the hydrogen atom, which the only atom for which an exact analytic solution to its Schrödinger equation is known. In heavier atoms, like carbon, nitrogen, and oxygen, the atomic orbitals involved in bonding are the 2s and 2p orbitals. These orbitals can be occupied in a hydrogen atom, but only when the electron is in an excited state. Hybrid orbitals are assumed to be mixtures of these atomic orbitals, superimposed on each other in various proportions. It provides a quantum mechanical insight to Lewis structures. Chemists use hybridization theory mainly in organic chemistry.
spx and sdx terminology
This terminology describes the weight of the respective components of a hybrid orbital. For example, in methane, the C hybrid orbital which forms each C-H bond consists of 25% s character and 75% p character and is thus described as sp3 (read as s-p-three) hybridised. Quantum mechanics describes this hybrid as an sp3 wavefunction of the form N[s + (√3)pσ], where N is a normalization constant (here 1/2) and pσ is a p orbital directed along the C-H axis to form a sigma bond. The p-to-s ratio (denoted λ in general) is √3 in this example, and N2λ2 = 3/4 is the p character or the weight of the p component.
For atoms forming equivalent hybrids with no lone pairs, there is a correspondence to the number and type of orbitals used. For example, sp3 hybrids are formed from one s and three p orbitals. However, in all other cases, there is no such correspondence. The two bond-forming hybrid orbitals of oxygen in water can be described as sp4, which means that they have 20% s character and 80% p character, but does not imply that they are formed from one s and four p orbitals. As a result, the amount of p-character is not restricted to integer values; i.e., hybridisations like sp2.5 are also readily described.
In general, for an atom with s and p orbitals forming hybrids hi and hj with included angle [math]\displaystyle{ \theta }[/math], the following holds: 1 + [math]\displaystyle{ \lambda }[/math]i[math]\displaystyle{ \lambda }[/math]j cos([math]\displaystyle{ \theta }[/math]) = 0. The p-to-s ratio for hybrid i is [math]\displaystyle{ \lambda }[/math]i2, and for hybrid j it is [math]\displaystyle{ \lambda }[/math]j2. The bond directed towards a more electronegative substituent tends to have higher p-character as stated in Bent's rule. In the special case of equivalent hybrids on the same atom, again with included angle [math]\displaystyle{ \theta }[/math], the equation reduces to just 1 + [math]\displaystyle{ \lambda }[/math]2 cos([math]\displaystyle{ \theta }[/math]) = 0. For example, BH3 has a trigonal planar geometry, three 120° bond angles, three equivalent hybrids about the boron atom, and thus 1 + [math]\displaystyle{ \lambda }[/math]2 cos([math]\displaystyle{ \theta }[/math]) = 0 becomes 1 + [math]\displaystyle{ \lambda }[/math]2 cos(120°) = 0, giving [math]\displaystyle{ \lambda }[/math]2 = 2 for the p-to-s ratio. In other words, sp2 hybrids.
An analogous notation is used to describe sdx hybrids. For example, the permanganate ion (MnO4-) has sd3 hybridisation with orbitals that are 25% s and 75% d.
Types of Hybridization
sp3 hybrids
Hybridisation describes the bonding atoms from an atom's point of view. That is, for a tetrahedrally coordinated carbon (e.g., methane CH4), the carbon should have 4 orbitals with the correct symmetry to bond to the 4 hydrogen atoms.
Carbon's ground state configuration is 1s2 2s2 2px1 2py1 or more easily read:
[math]\displaystyle{ C\quad \frac{\uparrow\downarrow}{1s}\; \frac{\uparrow\downarrow}{2s}\; \frac{\uparrow\,}{2p_x}\; \frac{\uparrow\,}{2p_y}\; \frac{\,\,}{2p_z} }[/math]
The carbon atom can utilize its two singly occupied p-type orbitals (the designations px py or pz are meaningless at this point, as they do not fill in any particular order), to form two covalent bonds with two hydrogen atoms, yielding the "free radical" methylene CH2, the simplest of the carbenes. The carbon atom can also bond to four hydrogen atoms by an excitation of an electron from the doubly occupied 2s orbital to the empty 2p orbital, so that there are four singly occupied orbitals.
[math]\displaystyle{ C^{*}\quad \frac{\uparrow\downarrow}{1s}\; \frac{\uparrow\,}{2s}\; \frac{\uparrow\,}{2p_x} \frac{\uparrow\,}{2p_y} \frac{\uparrow\,}{2p_z} }[/math]
As the additional bond energy more than compensates for the excitation, the formation of four C-H bonds is energetically favoured.
Quantum mechanically, the lowest energy is obtained if the four bonds are equivalent which requires that they be formed from equivalent orbitals on the carbon. To achieve this equivalence, the angular distributions of the orbitals change via a linear combination of the valence-shell (Core orbitals are almost never involved in bonding) s and p wave functions[2] to form four sp3 hybrids.
[math]\displaystyle{ C^{*}\quad \frac{\uparrow\downarrow}{1s}\; \frac{\uparrow\,}{sp^3}\; \frac{\uparrow\,}{sp^3} \frac{\uparrow\,}{sp^3} \frac{\uparrow\,}{sp^3} }[/math]
In CH4, four sp3 hybrid orbitals are overlapped by hydrogen's 1s orbital, yielding four σ (sigma) bonds (that is, four single covalent bonds) of the same length and strength.
sp2 hybrids
Other carbon based compounds and other molecules may be explained in a similar way as methane. For example, ethene (C2H4) has a double bond between the carbons.
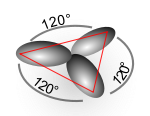
For this molecule, carbon will sp2 hybridize, because one π (pi) bond is required for the double bond between the carbons, and only three σ bonds are formed per carbon atom. In sp2 hybridization the 2s orbital is mixed with only two of the three available 2p orbitals:
[math]\displaystyle{ C^{*}\quad \frac{\uparrow\downarrow}{1s}\; \frac{\uparrow\,}{sp^2}\; \frac{\uparrow\,}{sp^2} \frac{\uparrow\,}{sp^2} \frac{\uparrow\,}{2p} }[/math]
forming a total of 3 sp2 orbitals with one p-orbital remaining. In ethylene (ethene), the two carbon atoms form a σ bond by overlapping two sp2 orbitals and each carbon atom forms two covalent bonds with hydrogen by s–sp2 overlap all with 120° angles. The π bond between the carbon atoms perpendicular to the molecular plane is formed by 2p–2p overlap. The hydrogen–carbon bonds are all of equal strength and length, which agrees with experimental data.
sp hybrids
The chemical bonding in compounds such as alkynes with triple bonds is explained by sp hybridization.
[math]\displaystyle{ C^{*}\quad \frac{\uparrow\downarrow}{1s}\; \frac{\uparrow\,}{sp}\; \frac{\uparrow\,}{sp} \frac{\uparrow\,}{p} \frac{\uparrow\,}{p} }[/math]
In this model, the 2s orbital mixes with only one of the three p-orbitals resulting in two sp orbitals and two remaining unchanged p orbitals. The chemical bonding in acetylene (ethyne) (C2H2) consists of sp–sp overlap between the two carbon atoms forming a σ bond and two additional π bonds formed by p–p overlap. Each carbon also bonds to hydrogen in a sigma s–sp overlap at 180° angles.
Hybridisation and molecule shape
Hybridisation helps to explain molecule shape:
| Classification | Main group | Transition metal[3] |
|---|---|---|
| AX2 |
|
|
| AX3 |
|
|
| AX4 |
|
|
| AX5 | - |
|
| AX6 | - |
|
Main group compounds with lone pairs
For main group compounds with lone electron pairs, the s orbital lone pair (analogous to s-p mixing in molecular orbital theory) can be hybridised to a certain extent with the bond pairs[5] to maximize energetic stability according to its Walsh diagram. This rationalisation is applied to explain deviations in ideal bond angles (i.e. only p orbitals used for bonding), most commonly in second and third period elements.
- Trigonal pyramidal (AX3E1)
- s-orbital can be hybridised with the three p-orbital bonds to give bond angles greater than 90°.
- E.g., NH3
- Bent (AX2E1-2)
- s-orbital lone pair can be hybridised with the two p-orbital bonds to give bond angles greater than 90°. The out-of-plane p-orbital can either be a lone pair or pi bond. If it is a lone pair, it results in inequivalent lone pairs contrary to the common picture depicted by VSEPR theory (see below).
- E.g., SO2, H2O
- Monocoordinate (AX1E1-3)
- s-orbital lone pair can be hybridised with the p-orbital bond. The two out-of-line p-orbitals can either be lone pairs or pi bonds. The p-orbital lone pairs are inequivalent from the s-rich lone pair.
- E.g., CO, SO, HF
Hybridisation of hypervalent molecules
Traditional description
In general chemistry courses and mainstream textbooks, hybridisation is often presented for main group AX5 and above, as well as for transition metal complexes, using the hybridisation scheme first proposed by Pauling.
| Classification | Main group | Transition metal |
|---|---|---|
| AX2 | - |
|
| AX3 | - |
|
| AX4 | - |
|
| AX5 |
|
|
| AX6 |
|
|
| AX7 |
|
|
| AX8 |
|
|
| AX9 | - |
|
However, such a scheme is now superseded as more recent calculations based on molecular orbital theory have shown that in main-group molecules the d component is insignificant, while in transition metal complexes the p component is insignificant (see below).
Resonance description
As shown by computational chemistry, hypervalent molecules can only be stable given strongly polar (and weakened) bonds with electronegative ligands such as fluorine or oxygen to reduce the valence electron occupancy of the central atom to below 8[6] (or 12 for transition metals). This requires an explanation that invokes sigma resonance in addition to hybridisation, which implies that each resonance structure has its own hybridisation scheme. As a guideline, all resonance structures have to obey the octet rule for main group compounds and the dodectet (12) rule for transition metal complexes.
| Classification | Main group | Transition metal |
|---|---|---|
| AX2 | - | Linear (180°) |
| 300px | ||
| AX3 | - | Trigonal planar (120°) |
| 400px | ||
| AX4 | - | Square planar (90°) |
| 400px | ||
| AX5 | Trigonal bipyramidal (90°, 120°) | Trigonal bipyramidal (90°, 120°) |
| ||
| AX6 | Octahedral (90°) | Octahedral (90°) |
| 300px | ||
| AX7 | Pentagonal bipyramidal (90°, 72°) | Pentagonal bipyramidal (90°, 72°) |
| ||
| AX8 | Square antiprismatic | Square antiprismatic |
|
| |
| AX9 | - | Tricapped trigonal prismatic |
|
Main group compounds with lone pairs
For hypervalent main group compounds with lone electron pairs, the bonding scheme can be split into two components: the "resonant bonding" component and the "regular bonding" component. The "regular bonding" component has the same description (see above), while the "resonant bonding" component consists of resonating bonds utilizing p orbitals. The table below shows how each shape is related to the two components and their respective descriptions.
| Regular bonding component (marked in red) | ||||
|---|---|---|---|---|
| Bent | Monocoordinate | - | ||
| Resonant bonding component | Linear axis | Seesaw (AX4E1) (90°, 180°, >90°) | T-shaped (AX3E2) (90°, 180°) | Linear (AX2E3) (180°) |
| 200px | 200px | 200px | ||
| Square planar equator | - | Square pyramidal (AX5E1) (90°, 90°) | Square planar (AX4E2) (90°) | |
| 300px | 300px | |||
| Pentagonal planar equator | - | Pentagonal pyramidal (AX6E1) (90°, 72°) | Pentagonal planar (AX5E2) (72°) | |
| 300px | 300px | |||
Clarifying misconceptions
VSEPR electron domains and hybrid orbitals are different
The simplistic picture of hybridisation taught in conjunction with VSEPR theory does not agree with high-level theoretical calculations[5] despite its widespread usage in many textbooks. For example, following the guidelines of VSEPR, the hybridization of the oxygen in water is described with two equivalent lone electron-pairs.[7] However, molecular orbital calculations give orbitals that reflect the C2v symmetry of the molecule.[8] One of the two lone pairs is in a pure p-type orbital, with its electron density perpendicular to the H–O–H framework.[9] The other lone pair is in an approximately sp0.8 orbital that is in the same plane as the H–O–H bonding.[9] Photoelectron spectra confirm the presence of two different energies for the nonbonded electrons.[10]
Exclusion of d-orbitals in main group compounds
In 1990, Magnusson published a seminal work definitively excluding the role of d-orbital hybridization in bonding in hypervalent compounds of second-row elements. This had long been a point of contention and confusion in describing these molecules using molecular orbital theory. Part of the confusion here originates from the fact that one must include d-functions in the basis sets used to describe these compounds (or else unreasonably high energies and distorted geometries result), and the contribution of the d-function to the molecular wavefunction is large. These facts were historically interpreted to mean that d-orbitals must be involved in bonding. However, Magnusson concludes in his work that d-orbital involvement is not implicated in hypervalency.[11]
Exclusion of p-orbitals in transition metal complexes
Similarly, p-orbitals have long been thought to be utilized by transition metal centers in bonding with ligands, hence the 18-electron description; however, recent molecular orbital calculations have found that such p-orbital participation in bonding is insignificant,[12] even though the contribution of the p-function to the molecular wavefunction is calculated to be somwhat larger than that of the d-function in main group compounds.
Hybridization theory vs. MO theory
Hybridization theory is an integral part of organic chemistry and in general discussed together with molecular orbital theory in advanced organic chemistry textbooks although for different reasons. One textbook notes that for drawing reaction mechanisms sometimes a classical bonding picture is needed with two atoms sharing two electrons.[13] It also comments that predicting bond angles in methane with MO theory is not straightforward. Another textbook treats hybridization theory when explaining bonding in alkenes[14] and a third[15] uses MO theory to explain bonding in hydrogen but hybridisation theory for methane.
Bonding orbitals formed from hybrid atomic orbitals may be considered as localized molecular orbitals, which can be formed from the delocalized orbitals of molecular orbital theory by an appropriate mathematical transformation. For molecules with a closed electron shell in the ground state, this transformation of the orbitals leaves the total many-electron wave function unchanged. The hybrid orbital description of the ground state is therefore equivalent to the delocalized orbital description for explaining the ground state total energy and electron density, as well as the molecular geometry which corresponds to the minimum value of the total energy.
There is no such equivalence, however, for ionized or excited states with open electron shells. Hybrid orbitals cannot therefore be used to interpret photoelectron spectra, which measure the energies of ionized states, identified with delocalized orbital energies using Koopmans' theorem. Nor can they be used to interpret UV-visible spectra which correspond to electronic transitions between delocalized orbitals. From a pedagogical perspective, hybridization approach tends to over-emphasize localisation of bonding electrons and does not effectively embrace molecular symmetry as does MO theory.
Other pages
Other websites
Notes
- ↑ Although sometimes taught together with the valence shell electron-pair repulsion (VSEPR) theory, valence bond and hybridization are in fact not related to the VSEPR model. Gillespie, R.J. (2004), "Teaching molecular geometry with the VSEPR model", Journal of Chemical Education, 81 (3): 298–304, doi:10.1021/ed081p298
References
- ↑ Pauling, L. (1931), "The nature of the chemical bond. Application of results obtained from the quantum mechanics and from a theory of paramagnetic susceptibility to the structure of molecules", Journal of the American Chemical Society, 53 (4): 1367–1400, doi:10.1021/ja01355a027
- ↑ McMurray, J. (1995). Chemistry Annotated Instructors Edition (4th ed.). Prentice Hall. p. 272. ISBN 0-13-140221-8
- ↑ Weinhold, Frank; Landis, Clark R. (2005). Valency and bonding: A Natural Bond Orbital Donor-Acceptor Perspective. Cambridge: Cambridge University Press. pp. 381–383. ISBN 0-521-83128-8.
{{cite book}}: CS1 maint: multiple names: authors list (link) - ↑ 4.0 4.1 Martin Kaupp Prof. Dr. (2001). ""Non-VSEPR" Structures and Bonding in d(0) Systems". Angew Chem Int Ed Engl. 40 (1): 3534–3565. doi:10.1002/1521-3773(20011001)40:19<3534::AID-ANIE3534>3.0.CO;2-#.
- ↑ 5.0 5.1 Weinhold, Frank. "Rabbit Ears Hybrids, VSEPR Sterics, and Other Orbital Absurdities" (PDF). University of Wisconsin. Retrieved 2012-11-11.
- ↑ David L. Cooper , Terry P. Cunningham , Joseph Gerratt , Peter B. Karadakov , Mario Raimondi (1994). "Chemical Bonding to Hypercoordinate Second-Row Atoms: d Orbital Participation versus Democracy". Journal of the American Chemical Society. 116 (10): 4414–4426. doi:10.1021/ja00089a033.
{{cite journal}}: CS1 maint: multiple names: authors list (link) - ↑ Petrucci R.H., Harwood W.S. and Herring F.G. "General Chemistry. Principles and Modern Applications" (Prentice-Hall 8th edn 2002) p. 441
- ↑ Levine I.N. “Quantum chemistry” (4th edn, Prentice-Hall) p. 470–2
- ↑ 9.0 9.1 Laing, Michael J. Chem. Educ. (1987) 64, 124–128 "No rabbit ears on water. The structure of the water molecule: What should we tell the students?"
- ↑ Levine p. 475
- ↑ E. Magnusson. Hypercoordinate molecules of second-row elements: d functions or d orbitals? J. Am. Chem. Soc. 1990, 112, 7940-7951.
- ↑ O’Donnell, Mark (2012). "Investigating P-Orbital Character In Transition Metal-to-Ligand Bonding" (PDF). Brunswick, ME: Bowdoin College. Retrieved 2012-09-16.
- ↑ Clayden, Jonathan; Greeves, Nick; Warren, Stuart; Wothers, Peter (2001). Organic Chemistry (1st ed.). Oxford University Press. p. 105. ISBN 978-0-19-850346-0.
- ↑ Organic Chemistry, Third Edition Marye Anne Fox James K. Whitesell 2003 ISBN 978-0-7637-3586-9
- ↑ Organic Chemistry 3rd Ed. 2001 Paula Yurkanis Bruice ISBN 0-13-017858-6